Solid Solutions

A pure metal consisting of only one type of atom is almost impossible. Practically impurities or foreign atoms always present in all materials.

UNIT - 1

Constitution of Alloys and Phase Diagrams

"It is difficult to say what is impossible, for the dream of yesterday

is the hope of today and the reality of tomorrow."

- Robert H. Goddard

SOLID SOLUTIONS

1. IMPURITIES IN SOLIDS

✓ A pure metal consisting of only one type of atom is almost impossible. Practically impurities or foreign atoms always present in all materials.

✓ Only very few metals are used in the pure or nearly pure form.

● For example, high-purity copper of 99.99% purity is used for electronic wires because of its very high electrical conductivity.

● High purity aluminium of 99.99% purity, also called superpure aluminium, is used for decorative purposes because it can be finished with a very bright metallic surfaces.

† Do you know? Even with today's sophisticated techniques, it is difficult to refine metals to a purity in excess of 99.9999%. At this level, on the order of 1022 to 1023 impurity atoms will be present in 1 m3 of material.

✓ However, the fact is that most engineering metals are intentionally added with other metals or non-metals to enhance/ produce increased strength, higher corrosion resistance, or other desired properties.

● For example, sterling silver is a 92.5% silver - 7.5% copper 2016 alloy. In normal atmospheric conditions, pure silver is highly corrosion resistant, but also very soft which is mostly undesirable. But adding 7.5% Cu makes the silver stronger, harder and hence more durable (without compromising its corrosion resistance property).

1. Alloys

✓ A metal alloy, or simply an alloy, is a mixture of two or more metals or a metal (metals) and a non-metal (non-metals).

✓ The element which is present in the largest proportion is called the base metal, and all other elements present are called yw alloying elements.

✓ The presence of alloying elements changes the physical, chemical and mechanical properties of the base metal to a considerable extent.

✓ The type and extent of change of properties depends on whether Tein the alloying elements are insoluble, dissolve in the base metal, or react with the base metal to form other phases.

SOLID SOLUTIONS

✓ A solid solution is the simplest type of alloy.

✓ A solution can be defined as a homogeneous mixture in which the atoms or molecules of one substance are dispersed at random into another substance.

✓ A solid solution may be defined as a solid that consists of two or more elements atomically dispersed in a single-phase structure.

✓ A solid solution is composed of two parts.

1. Solute: A solute is the minor part of the solution or the material which is dissolved.

2. Solvent: Solvent constitutes the major portion of the pitia solution.

Both the solute and the solvent can be solid, liquid or gas.

✓ A solid solution is formed when the solute atoms are added to the host material (solvent), without changing the existing crystal structure.

1. Solid Solution Vs Liquid Solution

✓ The concept of solid solution is analogous to that of the liquid solution.

✓ Fig.1.1 shows the formation of liquid solution of water and alcohol. The water-alcohol liquid solution is produced as the molecules intermix, and its composition is homogeneous throughout.

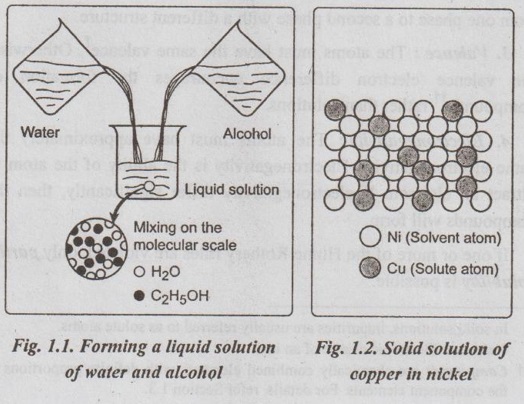
✓ Fig.1.2 shows a solid solution of copper and nickel atoms sharing the FCC crystal structure. Here nickel acts as a solute dissolving in the copper solvent.

✓ It can be noted from the Figs. 1.1 and 1.2 that similar to the liquid solution, the solid solution is also compositionally homogeneous; the impurity atoms* are randomly and uniformly dispersed within the solid.

2. Hume Rothery's Rules

(Factors Governing Solid Solubility)

To form an extensive solid solution (i.e., greater than 10 atomic percent soluble), the solute and solvent elements should obey the following general rules of Hume Rothery.

1. Size factor: The atoms must be of similar size, with less than a 15% difference in atomic radius (in order to minimise the lattice strain).

2. Crystal structure: The materials must have the same crystal structure. Otherwise, there is some point at which a transition occurs from one phase to a second phase with a different structure.

3. Valence: The atoms must have the same valence. Otherwise, the valence electron difference encourages the formation of tt compounds rather than solutions.

4. Electronegativity: The atoms must have approximately the same electronegativity. Electronegativity is the ability of the atom to attract an electron. If electronegativity differ significantly, then the compounds will form.

If one or more of the Hume Rothery rules are violated, only partial solubility is possible.

* In solid solutions, impurities are usually referred to as solute atoms.

† Valence : Electronic charge of an ion.

†† Compounds are chemically combined elements with definite proportions of the component elements. For details, refer Section 1.3.

3. Possibilities of Solid Solutions

There are three possible solid solutions based on the amount of their elements. They are:

1. Unsaturated solid solution: If the solvent is dissolving small amount of solute as well as at a given temperature and pressure, it is called unsaturated solid solution.

2. Saturated solid solution: If the solvent is dissolving limiting amount of solute, it is called saturated solid solution.

3. Supersaturated solid solution: If the solvent is dissolving more of solute than it should, under equilibrium, it is called supersaturated solid solution.

4. Types of Solid Solutions

Two kinds of solid solutions formed are:

1. Substitutional solid solutions

(a) Random, and (b) Ordered.

2. Interstitial solid solutions

1. Substitutional Solid Solutions

✓ When the solute atoms (impurities) substitute for parent solvent atoms in a crystal lattice, they are called substitutional atoms, and the mixture of the two elements is called a substitutional solid solution.

✓ In other words, in substitutional solid solution, the atoms of the solvent substitute for atoms of the solute in the lattice structure of the solvent.

✓ This type of solid solution is quite common among various hile metal systems.

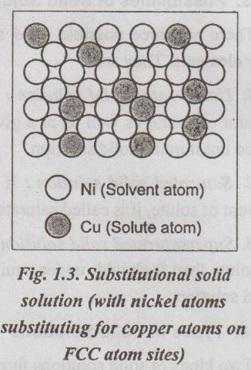
✓ Illustration: A Cu-Ni system, shown in Fig.1.3, is an example for a substitutional solid solution. These two elements are completely soluble in one another at all proportions. This system also satisfies all the Hume Rothery's rules that govern the degree of solubility, as below:

(i) The atomic radii for copper and nickel are 1.28 Å and 1.25 Å respectively;

(ii) Both the Cu and Ni have the FCC crystal structure;

(iii) The most common valencies are +1 for Cu and +2 for Ni; and 

(iv) The electronegativities of Cu and Ni are 1.9 and 1.8 novice respectively.

Thus, Cu-Ni system forms an extensive substitutional solid solution.

(a) Random (or Disordered) Substitutional Solid Solution

✔ In random substitutional solid solution, there is no order in the substitution of the two elements; the solute and solvent atoms are randomly distributed.

✔ In the formation of a substitutional solid solution, the solute atoms do not occupy any specific position but are distributed at random in lattice structure of the solvent. This alloy is said to be in a random or disordered condition.

✔ Fig.1.4 illustrates a random substitutional solid solution of copper-zinc system (i.e., brass). Here the crystal pattern is not altered.

(b) Ordered Substitutional Solid Solution

✔ If the solute and solvent atoms take up some preferred position, then the solution is called ordered substitutional solid solution or super lattice.

✔ Fig.1.5 shows an ordered substitutional solid solution.

✔ Diffusion (that takes place during cooling) tends to produce uniform distribution of solute and solvent atoms. Thus the solute atoms move into definite orderly positions in the lattice, as shown in Fig.1.5.

✔ Examples: Au-Cu and Cu2MnAl have ordered crystal structures.

2. Interstitial Solid Solution

✔ In interstitial solid solution, the solute atoms fit into the space between the solvent or parent atoms. These spaces or voids are called interstices.

✔ Interstitial solid solution can form only when one atom is much larger than another.

✔ The atoms which have atomic radii less than 1 Å are likely to form an interstitial solid solution. Such atoms are hydrogen (0.46 Å), carbon (0.77 Å), nitrogen (0.71 Å), and oxygen (0.6 Å).

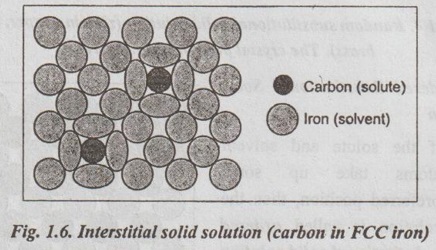
✔ Illustration: Fig.1.6 illustrates an interstitial solid solution that is formed by carbon in FCC γ iron just above 912°C. In this case, the atomic radius of carbon is 0.75 Å and that of 1.29 Å, and so there is an atomic radius difference of 42%.

✔ Like substitutional solid solutions, interstitial solid solutions also depend on size, valency, and electronegativity factors. But they do not depend on the type of crystal structure.