Two mark Questions and Answers: Transverse Loading on Beams and Stresses in Beam - Strength of Materials
TWO MARK QUESTIONS AND ANSWERS
1. Define Beam.
Beam is a structural member which is supported along the length and subjected to external loads acting transversely i.e., perpendicular to the centre line of the beam. Beam is sufficiently long as compared to the lateral dimensions.
2. What is meant by transverse loading on beams?
If a load is acting on the beam which is perpendicular to the centre line of it, then it is called transverse loading.
3. How to classify the beams according to its supports?
The beam may be classified according to the support as following:
(a) Cantilever beam
(b) Simply supported beam
(c) Over hanging beam
(d) Fixed beam and
(e) Continuous beam
4. What is cantilever beam?
A beam with one end free and the other end fixed is called cantilever beam.

5. What is simply supported beam?
A beam supported or resting freely on the supports at its both ends is called simply supported beam which is shown in Figure.

6. What is meant by overhanging beam?
If one or both of the end portions are extended beyond the support, then it is called overhanging beam.
7, What are the types of transverse load?
A beam may be subjected to the following types of transverse loads,
(a) Point or concentrated load.
(b) Uniformly distributed load.
(c) Uniformly varying loads.
8. What is meant by point or concentrated loads?
A load (W) which is acting at a point is called point load.
9. What is uniformly distributed load?
If a load which is spread over a beam in such a manner that rate of loading 'w' is uniform through out the length, then it is called as UDL.
10. Define shear force and bending moment at a section.
Shear Force: SF at any cross section is defined as the algebraic sum of all the forces acting either side of a beam.
Bending Moment: BM at a cross section is the algebraic sum of the moments of all the forces which are placed either side from that point.
11. What is meant by positive or sagging BM?
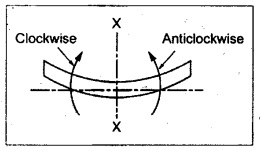
BM is said to be positive or sagging if the moment of the force in the left side of the beam is clockwise or right side of the beam is counter clockwise. Otherwise the beam tends to bend like concave manner.
12. What is meant by negative or hogging BM?
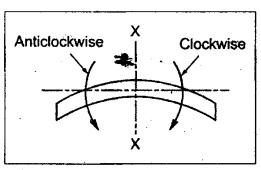
BM is said to be negative or hogging if the moment of the forces in the left side of the beam is counter clockwise or right side of the beam is clockwise. Otherwise the beam tends to bend like convex manner.
13. Draw the rough sketch of SF and BM for the beam given below.
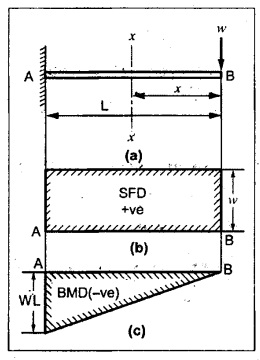
14. Draw the rough sketch of SF and BM for the beam given below.

15. What will be the SF and BM diagrams for the simply supported beam of lengh 'L' subjected to central point load 'W"?
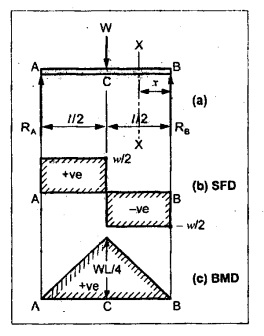
16. Draw the SF and BM diagrams for the simply supported beam of length ‘L' subjected to UDL of w/m length throughout its length.

17. When will be the BM is maximum?
BM will be maximum when the SF changes its sign.
18. In simply supported beam, the BM at the support is always―――.
Zero.
19. What is meant by reaction?
The upward force provided by the supports to balance the vertical downward load is called reaction.
20. What is the nature of curve when the beam carrying UDL?
The curve follows parabolic relation or parabolic curve.
21. What are the SF and BM diagram for the beam given below?
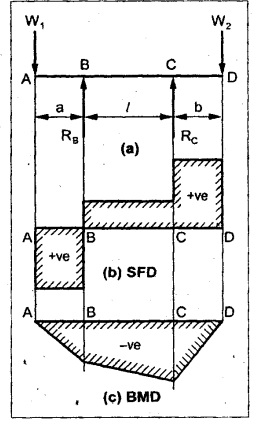
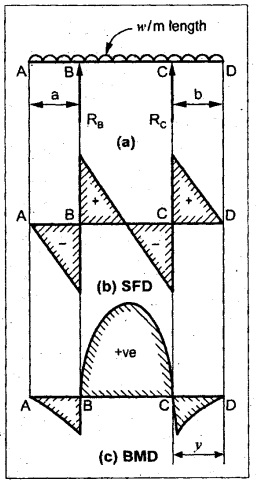
22. Draw the SFD and BMD for the overhanging beam carrying UDL throughout its length and supported at 'a' distance from end A and 'b' distance from end B.
23. The BM at the support of the overhanging beam is always zero - True/False.
False. Since in overhanging beam, the BM always zero at both the ends.
24. Draw the BMD for cantilever beam subjected to an anticlockwise moment at its free end.
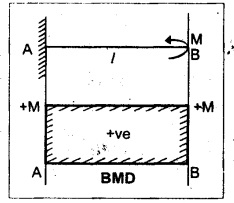
BM calculation:
BM at B = +M
(Since +ve for counter clockwise moment)
BM at A = +M
(BM at both the ends A are same)
25. Define the term point of contraflexure.
The point where the BM changes its sign or zero is called the point of contraflexure.
26. What are SF and BM diagrams?
SF diagram shows the variation of forces along the length of the beam.
BM diagram shows the variation of bending moment along the length of the beam.
27. Draw the SF and BM diagram for a cantilever beam of span 'L' carrying a point load 'W' at a distance of 'a' from free end.
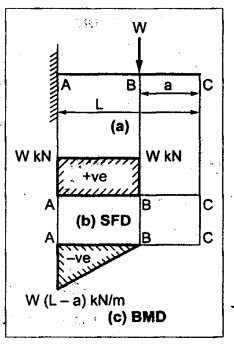
SF calculation:
SF at C = 0
SF at B = W kN
SF at A = W kN
BM calculation:
BM at C = 0
BM at B = 0.
BM at A = w × (L - a) kN - m
28. Write the relation between SF and BM.
The rate of change of BM is equal to the SF at the section,
dM /dx = -F
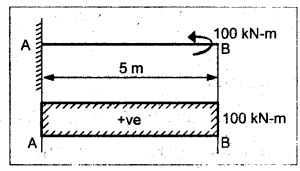
29. A cantilever beam of length 5m is acted upon by a force couple of moment 100 kNm at the free end. What is the bending moment at the fixed end?
BM calculation:
BM at B = + 100 kN-m
BM at A = + 100 kN-m
(+ve for counter clockwise moment)
[Since, couple is always independent of its span]
30. A cantilever beam of length 6m is acted upon by a concentrated load of 10 kN at 2 m from the free end. Calculate the SF and BM at the free end.
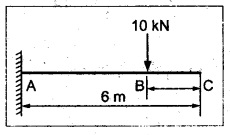
SF calculation:
SF at C = 0
SF at B = 10 KN
SF at A = 10 kN
BM calculation:
BM at C = 0
BM at B = 0
BM at A = 10 × 4 = 40 kN-m
⸫ The SF and BM at the free end are zero.
31. The maximum BM in a SSB of span ‘L' subjected to UDL of w over the entire span is ―――.
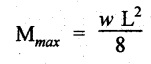
32. In SSB, how do you locate the point of maximum bending moment.
The BM is maximum when the SF changes its sign or zero. Write the SF equation at that point and equating it to zero we can find out the distance 'x' from one end. Then find the maximum BM at that point by taking moment of all forces left or right from that point.
33. A cantilever of span 4m is subjected to a UDL of 20 kN/m over its entire length. Sketch the BMD for the beam.
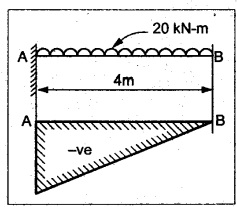
BM at B = 0
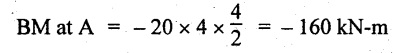
34. A cantilever beam of span 6m is subjected to a point load of 10 kN/m at the free end. What is the maximum BM?
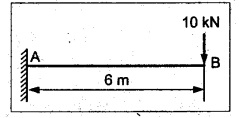
BM at A = 0
BM at B = 10 × 6
= 60 kN-m
⸫ The maximum BM = 60 kN-m
35. A beam AB of span 6m is subjected to a UDL of 10 kN/m over the entire span. What is the maximum BM?
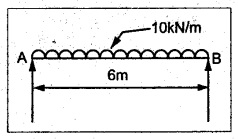
For this case, BM is maximum at mid span of the beam.
Mmax = 10 × 3 × 3/2
= 45 kN-m
36. Calculate the BM at the fixed end of a cantilever beam subjected to a point load of 10 kN at 3m from fixed end.
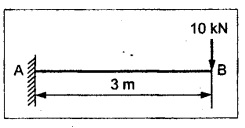
BM at A = 10 × 3
= 30 kN-m
The BM at fixed end is 30 kN-m.
Two mark Questions and Answers: Transverse Loading on Beams and Stresses in Beam - Strength of Materials
TWO MARK QUESTIONS AND ANSWERS
Theory of simple bending
1. Write down the bending equation.
The bending equation is 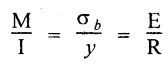
Where,
M - Bending moment
I - Moment of inertia of the section
σb - Bending stress at that section
y - Distance from the neutral axis
E - Young's modulus of the material
R - Radius of curvature of the beam
2. What are 'neutral axis' and 'neutral plane'?
Neutral plane: The surface in which there are no longitudinal stresses acting is called neutral plane or layer. In such planes there is neither compression nor expansion takes place due to transverse load.
Neutral axis: A trace of this plane on any transverse plane of the beam is called the neutral axis.
3. What are the assumptions made in the theory of bending?
(i) The material is perfectly homogeneous and isotropic. It obeys Hooke's law.
(ii) The value of Young's modulus is the same in tension as well as in compression.
(iii) The radius of curvature of the beam is very large compared to the cross section dimensions of the beam.
(iv) The resultant force on a transverse section of the beam is zero.
4. Define 'section modulus'.
It is the ratio of moment of inertia of the section to the distance of plane from neutral axis.

5. What is moment of resistance of the section?
It is the product of section modulus and the bending stress at that section.
M = σb × Z
6. What is a flitched beam? Why it is used?
A beam which is constructed by two different materials is called flitched beam or composite beam.
It is mainly used to reinforce the material which has lower strength and reduce the cost.
7. State the theory of simple bending.
If a beam is bend only due to application of constant bending moment and not due to shear, then it is called simple bending.
8. A beam subjected to a bending stress of 5 N/mm2 and the section modulus is 3530 cm3. What is the moment of resistance of the beam?
σb = 5 N/mm2;
Z = 3530 cm3 = 3530 × 103 mm3
Moment of resistance. M = σb × Z = 5 × 3530 × 103
= 17.65 × 106 N-mm
9. What is the section modulus for a circular and a hollow circular section?

Where, D - outer diameter, d - inner diameter.
10. The section modulus of a circular section of diameter 30 mm is ――
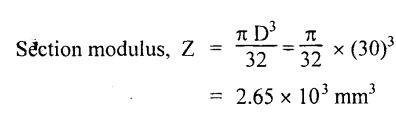
11. Is bending stress a direct stress or shear stress?
Direct stress
Two mark questions & answers on shear stress distribution : Transverse Loading on Beams and Stresses in Beam - Strength of Materials
TWO MARK QUESTIONS & ANSWERS ON SHEAR STRESS DISTRIBUTION
1. What types of stresses are caused in a beam subjected to a constant shear force?
Vertical and horizontal shear stress.
2. State the main assumptions while deriving the general formula for shear stresses?
1. The material is homogeneous, isotropic and elastic.
2. The modulus of elasticity in tension and compression are same.
3. The shear stress is constant along the beam width.
4. The presence of shear stress does not affect the distribution of bending stress.
3. Define Shear stress distribution.
The variation of shear stress along the depth of the beam is called shear stress distribution.
4. Write down the expression for shear stress distribution in a beam subjected to shear force F.
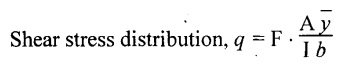
5. Write the formula to find the shear stress distribution for a rectangular beam section and sketch the shear stress distribution.
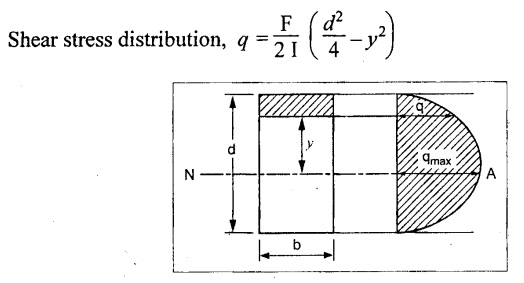
5. What is the ratio of maximum shear stress to the average shear stress for the rectangular section?
qmax = 1.5 × qave
6. Draw the shear stress distribution in the solid circular section.

7. What is the ratio of a maximum shear stress to the average shear stress in the case of solid circular section?
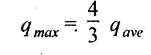
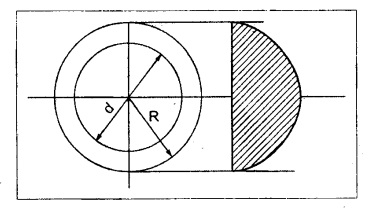
8. Sketch the shear stress distribution in a beam made of hollow circular section.
9. What is the maximum value of shear stress for triangular section?
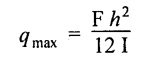
10. Draw the shear stress distribution in the case of triangular section and where the maximum shear stress occurs.
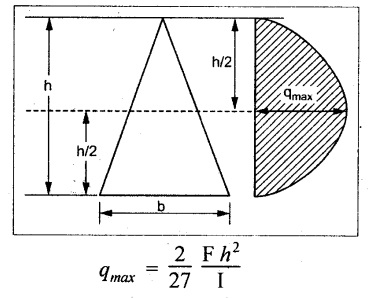
Maximum shear stress occurs at half of the height of the triangle.
11. Draw the shear stress distribution of I-symmetrical section.

12. What is the shear stress distribution value of flange portion of the I-section?

where, y - distance of flange portion from N.A.
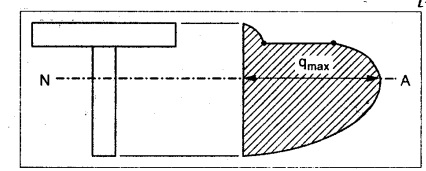
13. Draw the shear stress distribution in the case of ‘T’ - section.
14. What is the value of maximum shear stress in a rectangular cross section?
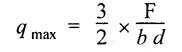
No comments:
Post a Comment