Unit Quantities

 The following are the three important unit quantities which must be studied under unit head:

UNIT QUANTITIES

In order to predict the behaviour of a turbine working under varying conditions of head, speed, output and gate opening, the results are expressed in terms of quantities which may be obtained when the head on the turbine is reduced to unity. The conditions of the turbine under unit head are such that the efficiency of the turbine remains un affected. The following are the three important unit quantities which must be studied under unit head:

(1) Unit speed

(2) Unit discharge and

(3) Unit power

1. Unit Speed

It is defined as the speed of the turbine working under a unit head (i.e, under a head of 1 m) It is denoted by 'Nu'. The expression for unit speed (Nu) is obtained as.

Let N = Speed of a turbine under a head H

H = Head under which a turbine is working

u = Tangential velocity

The tangetial velocity, absolute velocity of water and head on the turbine are related as

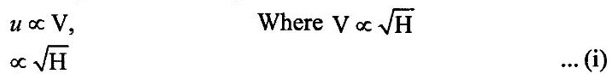
Also tangential velocity (u) is given by

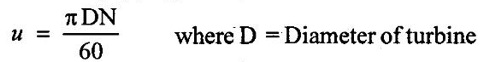
For a given turbine, the diameter (D) is constant

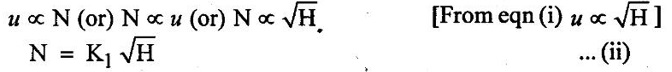
Where K1 is a constant of proportionality.

If head on the turbine become unity, the speed becomes unit speede

H = 1, N = Nu

Substituting these values in eqn (ii)

Substituting the value of K1 in eqn (iii)

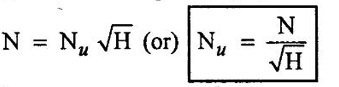
2. Unit Dischare

It is defined as the discharge passing through a turbine, which is working under a unit head It is denoted by Qu. The expression for unit discharge is given as:

H = head of water on the turbine

Q = Discharge passing through turbine when head is H on the turbine. 

a =Area of flow of water

The discharge passing through a given turbine under a head 'H' is given by

Q = Area of flow × velocity

But for a turbine, area of flow is constant and velocity is propertional to √H.

Where K2 is constant of proportionality

If H = 1, Q = Qu

Substating these values in eqn (i)

Qu = K2 √1.0 = K2 

Substituting the value of K2 in eqn (i)

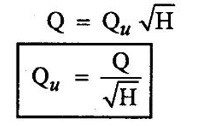
3. Unit Power

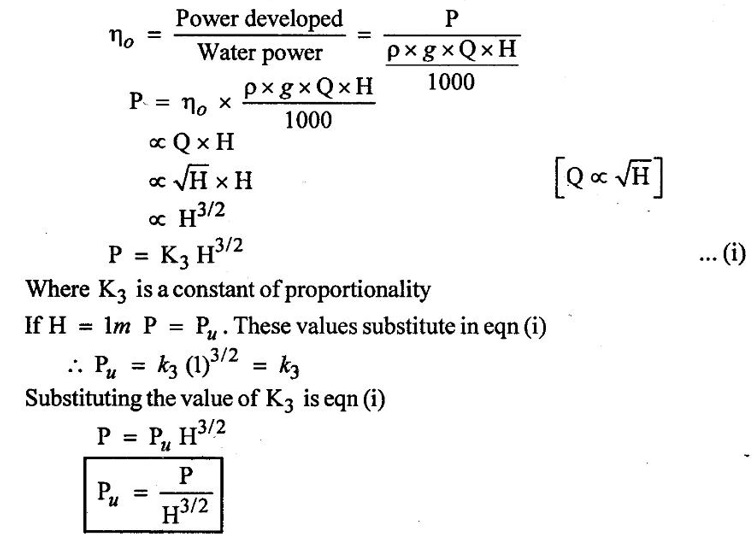
It is defined as the power developed by a turbine, working under a unit head. It is denoted by 'Pu'. The expression for unit power is obtained as

H = Head of water on the turbine

P = Power developed by the turbine under a head

Q = Discharge through turbine under a head H

The overall efficiency (η0) is given as

4. Use of unit Quantities (Nu, Qu, Pu)

If a turbine is working under different heads the behaviour of the turbine can be easily known from the values of the unit quantities i.e., from the values of unit speed, unit discharge and unit power

H1 H2 ... are the heads under which a turbine works

N1 N2 ... are the corresponding speeds

Q1 Q2 ... are the discharge, and

P1 P2 ... are the power developed by the turbine using equation.

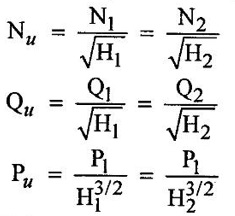
Hence if the speed, discharge and power developed by a turbine under a head are known, then by using above eqns the speed discharge and power developed by the same turbine under a different head can be obtained easily.

5. Solved Examples based on Unit Quantities

Example - 49

A turbine develops 9000 Kw when running at 100 rpm. The head on the turbine is 30 m. If the head on the turbine is reduced to 18m, determine the speed and power developed by this turbine.

Given data:

Power developes P1 = 9000 Kw

Head H1 = 30 m

Speed N1 = 100 rpm

Let for ahead H2 = 18m

To find:

Power P2 = ?

Speed N2 = ?

Solution:

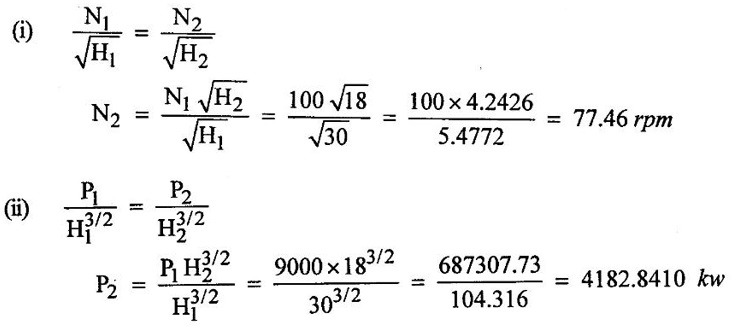
Result:

N2 = 77.46 rpm;

P2 = 4182.84 kw

Example - 50

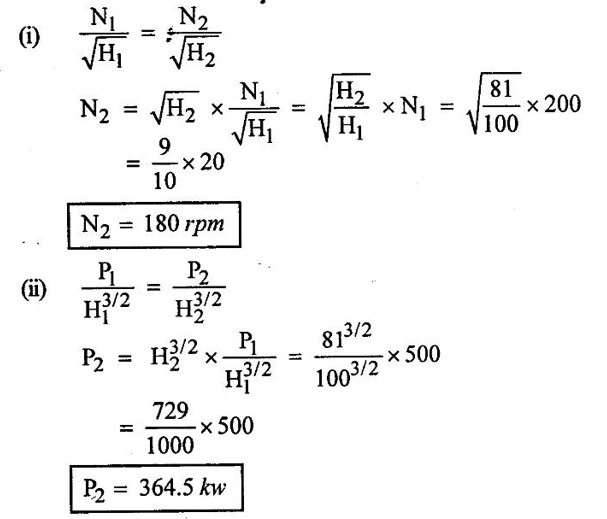
A turbine developed 500 kw power under head of 100 meters at 200 rpm. What would be its normal speed and output head of 81 meters?

Given data:

Power P1 = 500 kw

Speed N1 = 200 rpm

Head H1 = 100 m

For a new head H2 = 81 m

To find:

Normal Speed N2 = ?

Normal Power P2 = ?

Solution:

Result:

N2 = 180 rpm;

P2 = 364.5 kw

Example - 51

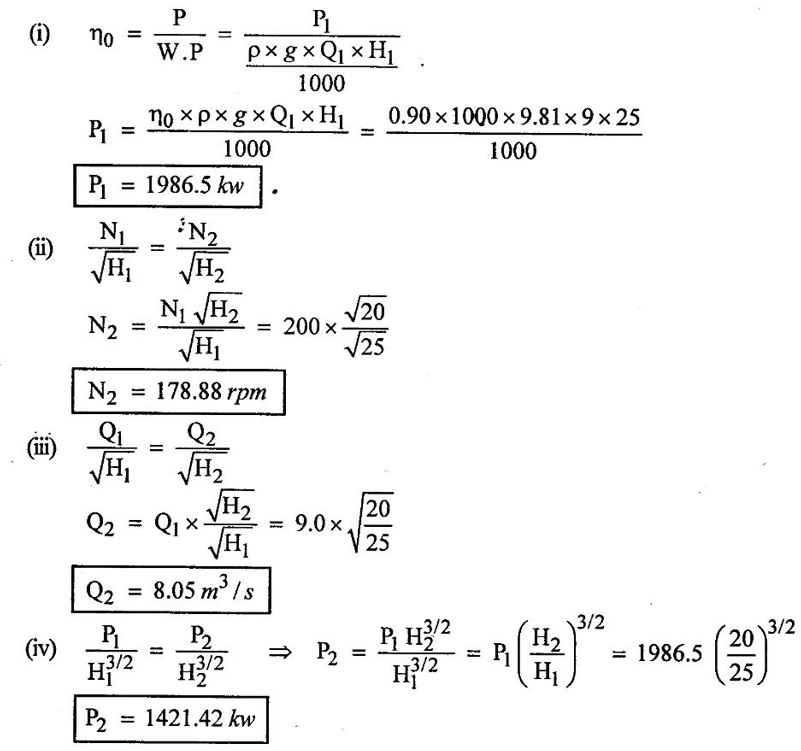
A turbine is to operate under a head of 25m at 200 rpm. The discharge is 9 cumec. If the efficiency is 90% determine the performance of the turbine under a head of 20m.

Given data:

Head on turbine H1 = 25m 

Discharge Q1 = 9m3/s

Speed N1 = 200 rpm

Overall efficiency η0 = 90% (or) 0.90

Performance of a turbine under a head H2 = 20m

To find:

The speed N2 =?

Discharge Q2 = ?

Power P2 =?

Solution:

Result: 

N2 = 178.88 rpm

Q2 = 8.05 m3/s

P2 = 1421.42 kw

Example - 52

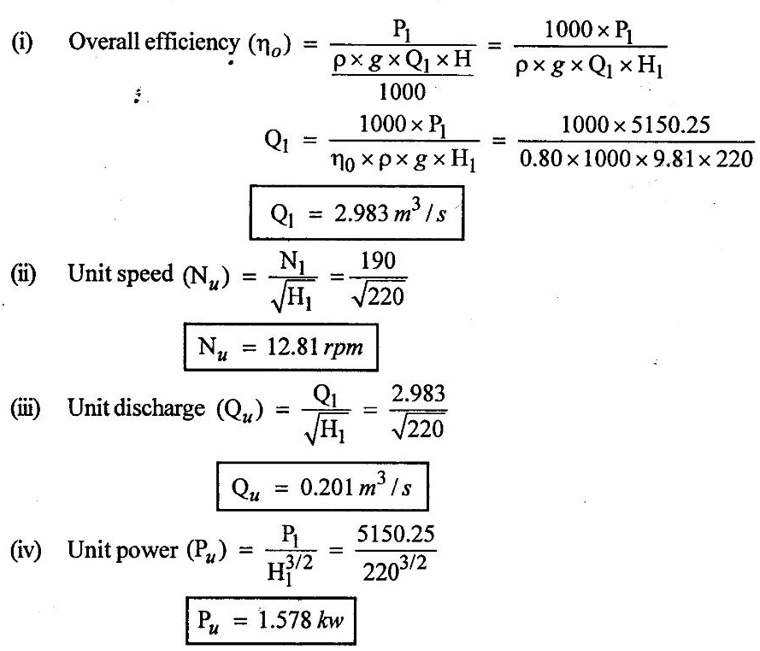
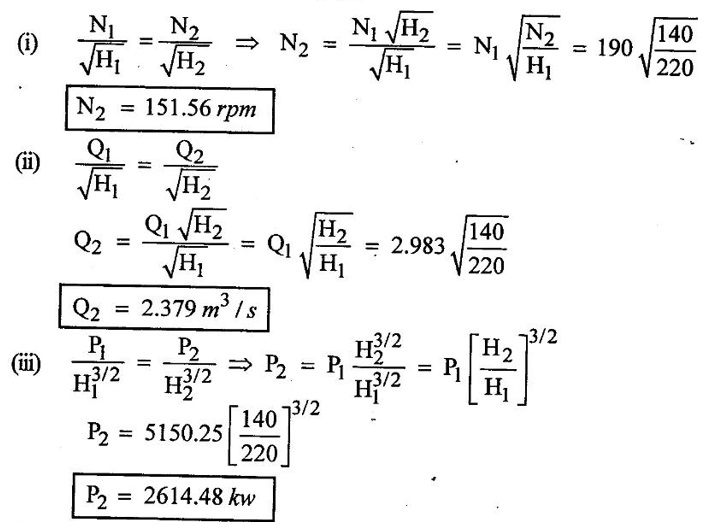
A pelton wheel is revoling at a speed of 190 rpm and developed 5150.25 kw when working under a head of 220m with an overall efficiency of 80% Determine unit speed, unit discharge and unit power. The speed ratio for the turbine is given as 0.47. Find the speed, discharge and power when this turbine is working under a head of 140m.

Given data:

Speed N1 = 190 rpm 

Head H1 = 220m 

Speed ratio = 0.47

Power P1 = 5150.25 kw

Over all efficiency η0 = 80% = 0.80 

New head of water H2 = 140m

To find:

(i) Nu, Qu, and Pu head of 220 m 

(ii) N2, Q2, and P2 head of 140 m

Solution:

Case-I Working under a head of 220 m

Case-II When the turbine is working under a new head of 140m

Result:

Case-head of 220 m: Nu = 12.81 rpm, Qu = 0.201 m3/s, Pu, = 1.578 kw

Case-II head of 140 m: N2 = 151.56 rpm, Q2 = 2.379 m3 / s, P2 = 2614.48 kw