Stability of a Submerged Body

 The stability of a submerged body is determined by the relative position of the centre of gravity G and centre of buoyancy B of the body.

STABILITY OF A SUBMERGED BODY

The stability of a submerged body is determined by the relative position of the centre of gravity G and centre of buoyancy B of the body. If G is located below B, the body remains in stable equilibrium. IfG is above B, then the body is in unstable equilibrium. If G coincides with B, the body is in neutral equilibrium.

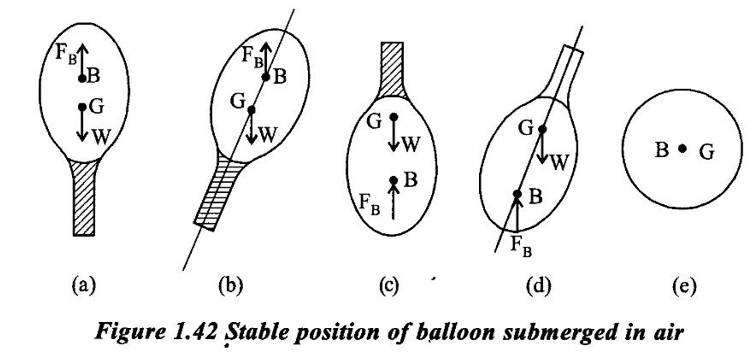
Consider a balloon submerged in air an shown in figure or a submarine fully submerged in water. If the lower passion of the body contains heavier material, the centre of gravity remains below its centre of buoyance B as in figure 1.42 (a).

Since the body is in equilibrium, the weight of the body w, acting at G is equal to the buoyancy force FB acting at centre of buoyancy B. Now if the body is given a clock wise angular displacement shown in figure 1.42 (b) which will bring the body to its original passion. This is the stable equilibrium condition of the body.

On the other, if the upper passion of the body contains heavier material, G remains above B as shown in figure 1.42 (c).

For equilibrium w = FB and if the body is given a clock wise angular displacement, then w and FB constitute a clockwise couple as shown in figure 1.42 (d). While will further tend to overturn in the clockwise direction.

The body cannot return to its original position and the body is in unstable equilibrium. Now, if the weight of the body is such that B coincides with G and FB = w, as shown in figure 1.42 (e). It is in a neutral state of equilibrium.