Buoyancy

 When a body is immersed partially or fully in a fluid it experiences a vertical upward force.

BUOYANCY AND FLOATATION

1. Introduction

When a body is immersed partially or fully in a fluid it experiences a vertical upward force. This upward vertical force acting on a floating of submerged body is known as buoyancy force. The buoyance force is equal to the weight of the fluid displaced by the body or equal to the loss of weight of the body when it is weighed in the liquid. This buoyance force acts through the centre of gravity of the displaced fluid and this point is called the centre of buoyance.

The stability of any floating of submerged body is the tendency of the body when it is slightly disturbed to return to or to go away from its original position. The body in a static equilibrium may or may not retain its original position when its slightly disturbed. The body is stable only or it returns to original position when its centre of stable floating body is slightly tilted lying on the normal axis of the body which is called the meta centre of the body.

2. Archimedes Principle and Centre of Buoyancy

Archimedes law states that whenever a solid body is immersed Wholly or partly in a liquid it is acted by a force equal to the weight of the liquid displaced by body in the opposite direction of gravitational force of the body.

Buoyant force = Loss of weight of the body (or)

Weight of the fluid displaced by the body.

Consider a body immersed in the liquid as shown in figure 1.39. Consider an elemental cylinder whose cross-sectional after dA and height of y and located of a height h shown the free surface of work.

i) Force acting on the elemental cylinder at point 1

F1 = p1 dA 

F1 = w× h • dA

ii) Force acting on the elemental cylinder of point 2

F2 = p2 dA

F2 = (w + h) dA

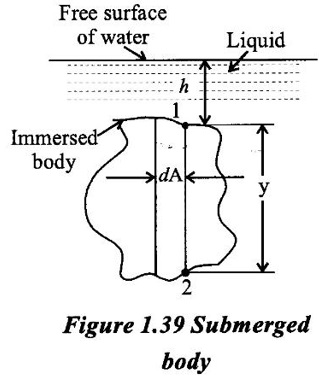
iii) Net force acting on the elemental cylinder

dF = F2 – F1

= w(h + y) dA wh dA

= wh dA + wy dA – wh dA

= wy dA

= w dỤ             (dU = y.dA volume of cylinder)

iv) Total force acting on the body (Fb)

Fb = ΣwdU = w⋅U

Where U is the total volume of the body. This force Fb acting on the body from the down is known as buoyancy force.

Anybody existing in the liquid the forces acting on the body are its weight (w) and buoyancy force (Fb). Whether the body sinks of floats depends upon the magnitudes of W and Fb.

a) If w > Fb then the body sinks and completely seated in bottom surface of the reservoir.

b) If w = Fb then the body floats touting its top to the free surface of water.

c) If w < Fb1 then the body floats of partially submerged in a fluid.

3. Applications of Buoyancy

1) The float valves for controlling the level of water in overhand storage tanks and cisterns work on the principle of buoyancy.

2) Determining the specific gravity of liquids. The hydrometers are working on the principle of buoyancy.

3) Surfacing and submerging of submarine is achieved by decreasing and increasing the weight of the submarine. Submarine has ballast tanks when these tanks are filled up, submarine goes down in sea water and when water is pumped out from the ballast tanks, the submarine surfaces on the surface.

4) Determining the volume of anybody, the loss of weight of anybody is equal to weight of the displaced volume of liquid. The volume displaced is equal to the volume of the body.

5) Life jacket for ships and rubber rafts for aeroplanes flying over the ocean work on the principle of buoyancy. These develop keep increasing the volume of displaced water.