Diamond Cubic Unit Cell

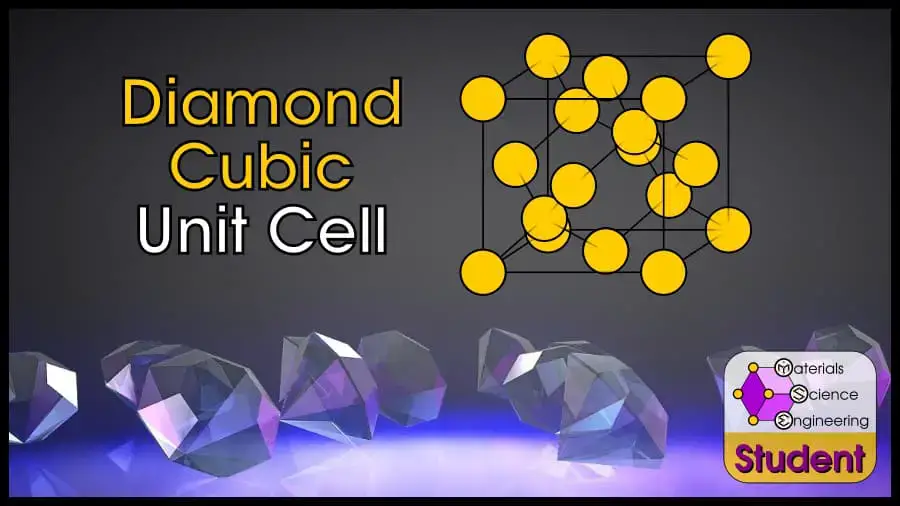
Diamond cubic (abbreviated DC in this article) is one of the advanced crystal structures (because it doesn’t correlate 1-1 to a Bravais lattice), so if you’re searching for information about it, I’m assuming you’re a somewhat advanced student in materials science. I’ll give you the facts quickly and with little explanation. If you are new to materials science and don’t know what something means, you can check out my articles on the FCC, BCC, or HCP crystals, which explain terminology more slowly.

The diamond cubic crystal structure is based on the face-centered cubic Bravais lattice (you can imagine it as two FCC unit cells, offset by ¼). There are 8 atoms per unit cell, and each atom is tetrahedrally coordinated so that it has 4 nearest neighbors. DC is a famously strong crystal structure, and is the structure of diamond. The diamond cubic cell belongs to space group 227 or $Fd\bar{3}m$ , Strukturbericht A4, and Pearson symbol cF8. C (diamond) is the prototype for DC.

The Diamond Cubic (DC) unit cell can be imagined as a cube with an atom on each corner, each face, and the (¼, ¼, ¼), (¾, ¾, ¼), (¼, ¼, ¾), and (¼, ¾, ¾) positions. DC has 8 atoms per unit cell, lattice constant a = 8R/√3, Coordination Number CN = 4, and Atomic Packing Factor APF = 34%.

Outline

Common Examples of Diamond Cubic Materials

The most notable material with a diamond cubic structure is, of course, carbon (in diamonds).

The diamond cubic structure is tetrahedrally coordinated, which means that each atom has 4 bonds. That’s why the other elements that form diamond cubic crystals are also in group 14.

Silicon, germanium, and α-tin can form the diamond cubic structure.

When there are two elements in this structure, the crystal is called “zincblende.” Silicon carbide, germanium arsenide, and other compounds may form the zincblende structure.

Diamond Cubic Coordination Number

Each atom in a diamond cubic crystal is tetrahedrally bonded to 4 other atoms, so there are 4 nearest neighbors (NNs).

In other words, the coordination number (CN) is 4. The NN distance is $\frac{\sqrt{3}}{4}a$ , and the next-nearest neighbor (NNN) distance is $\frac{\sqrt{2}}{2}a$ , where a is the lattice constant (I’ll prove this below).

Diamond Cubic Lattice Constants

Using the standard hard sphere model, nearest-neighboring atoms touch–that means they have a distance of $2r$ , where $r$ is the radius of the atoms.

We know that the closest 2 atoms are translated by ¼ of the lattice parameter. If one atom is at (0,0,0), then the closest atom is at (¼,¼,¼) times the lattice parameter.

We could solve this with a series of Pythagorean Theorems from different perspectives, like I did when calculating the lattice parameter for a BCC unit cell, but this is an advanced topic.

Let’s just use the distance formula in 3D. Starting at the atom in (0,0,0), the nearest atom is $\frac{1}{4}a$ in the x-direction, $\frac{1}{4}a$ in the y-direction, and $\frac{1}{4}a$ in the z-direction.

In other words $2r=\sqrt{(\frac{1}{4}a)^2+(\frac{1}{4}a)^2+(\frac{1}{4}a)^2}$

Solving this gives $\frac{8}{\sqrt{3}}r$ .

If you wanted to describe the diamond cubic crystal with math, you would describe the cell with the vectors:

$a_{1}=\frac{a}{2}\hat{y}+\frac{a}{2}\hat{z}$
$a_{2}=\frac{a}{2}\hat{x}+\frac{a}{2}\hat{z}$
$a_{3}=\frac{a}{2}\hat{x}+\frac{a}{2}\hat{y}$

And basis

$b_{1}=-\frac{1}{8}\hat{a_1}-\frac{1}{8}\hat{a_2}-\frac{1}{2}\hat{a_3}=-\frac{1}{8}\hat{x}-\frac{1}{8}\hat{y}-\frac{1}{2}\hat{z}$
$b_{2}=\frac{1}{8}\hat{a_1}+\frac{1}{8}\hat{a_2}+\frac{1}{2}\hat{a_3}=\frac{1}{8}\hat{x}+\frac{1}{8}\hat{y}+\frac{1}{2}\hat{z}$

Diamond Cubic Atomic Packing Factor

The atomic packing factor (APF) is the volume taken by atoms, divided by the volume taken by the whole unit cell. Since the diamond cubic crystal has a cubic volume that is directly related to the atomic radius, all diamond cubic crystals will have the same APF.

If you count the portions of the atoms inside the unit cell, there are 8 atoms on each corner which contribute ⅛ of their volume, 6 atoms on each face which contribute ½ of their volume, and 4 atoms inside which contribute their full volume. Thus, there are 8 atoms per unit cell.

The volume of a sphere is $\frac{4}{3}\pi r^3$ . We previously established that the area of the whole cube is $a^3$ , and since $a=\frac{8}{\sqrt 3}r$ , the volume of the cube is $(\frac{8}{\sqrt 3}r)^3$ .

$APF=\frac{8\cdot\frac{4}{3}\pi r^3}{(\frac{8}{\sqrt{3}}\pi r)^3}=\frac{\pi \sqrt{3}}{16}=34\%$

As you can see, diamond cubic crystals have 34% packing. That is abysmal, and is even lower than simple cubic, which is so unstable that it only exists in one element at room temperature and pressure.

Why does the diamond cubic occur at all if its packing factor is so low, and why is the strongest natural substance, diamond, have this crystal structure?

In the case of diamond, I would say that it exhibits strength despite its crystal structure. Carbon has 4 covalent bonds, which basically forces a tetragonal arrangement. These covalent bonds are very strong, which is why diamond is strong. If you could somehow get these same covalent bonds but in a higher-packed crystal structure like FCC, this FCC carbon should be much stronger than diamond.

Silicon, germanium, and alpha-tin also exhibit the diamond cubic structure, and they don’t display any special strength. In fact, when tin changes from beta-tin (room temperature) to alpha-tin (cold), the diamond cubic tin essentially disintegrates into powder (allegedly, this phase transformation and the fact that Napoleon’s troops wore jackets with tin buttons contributed to his failed invasion of Russia).

Primitive Diamond Cubic Unit Cell

Advanced topic, click to expand!

The diamond cubic cell that I have shown you is a conventional unit cell, not a primitive unit cell. This conventional cell has advantages because it is highly symmetric and easy for humans to understand.

However, when dealing with mathematical descriptions of crystals, it may be easier to describe the unit cell in the smallest form possible. The smallest possible unit cell is called the primitive cell. If you are interested in primitive cells, you can read all about them in this article.

The DC primitive cell looks like this:

Here are the primitive vectors for the FCC unit cell.

$a_{1}=\frac{a}{2}\hat{y}+\frac{a}{2}\hat{z}$
$a_{2}=\frac{a}{2}\hat{x}+\frac{a}{2}\hat{z}$
$a_{3}=\frac{a}{2}\hat{x}+\frac{a}{2}\hat{y}$

And basis

Final Thoughts

The diamond cubic crystal structure is an incredibly inefficient way to pack atoms, but it forms in atoms that form tetrahedral (4-way) bonds, like carbon. Although each atom is bonded only 4 times (instead of 12 as in close-packed structures), these bonds may each be very strong in the case of covalent-bonded elements like diamond carbon. On the other hand, when metallic bonded gray tin arranges in a diamond cubic structure, it becomes very weak.

VEERAPANDIAN.K , Assistant Professor

Pages

Friday, November 17, 2023